Главная страница | Контакты | Случайная страница Автомобили Астрономия Биология География Дом и сад Другие языки Другое Информатика История Культура Литература Логика Математика Медицина Металлургия Механика Образование Охрана труда Педагогика Политика Право Психология Религия Риторика Социология Спорт Строительство Технология Туризм Физика Философия Финансы Химия Черчение Экология Экономика Электроника

PROBLEM SET

Numerical Differentiation.

Example 1. Find at x = 0.1 from the following table:

Sol. Take a = 0.1. The difference table is:

Here h = 0.1 and y 0 = 0.9975

Example 2. The table given below reveals the velocity ‘v’ of a body during the time ‘t’ specified. Find its acceleration at t = 1.1.

Sol. The difference table is:

Let a = 1.1,

v 0 = 47.7 and h = 0.1

Acceleration at t = 1.1 is given by

Hence the required acceleration is 45.1667.

Example 3. Find f ′ (1.1) and f ″ (1.1) from the following table:

Sol. Since we are to find and for non-tabular value of x, we proceed as follows:

Newton’s forward difference formula is

(1)

where

(2)

Differentiating eqn. (2) with respect to u, we get

(3)

Differentiating eqn. (2) with respect to x

(4)

Also, at x = 1.1,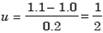

The forward difference table is as follows:

From eqn. (4),

(5)

At x = 1.1, we get

Differentiating eqn. (44), with respect to x, we get

At x = 1.1, we get

PROBLEM SET

1. Given that

Find and at (i) x = 1.1 (ii) x = 1.6.

2. Find first and second derivatives of the function tabulated below at x = 0.6

3. Find y ′(0) and y ″(0) from the given table:

4. Find y ′(1.5) and y ″(1.5) from the following table:

5. Given the following table of values of x and y:

Find and at (i) x = 1 (ii) x = 1.25 (iii) x = 1.15.